H.S.Math Teaching Ideas: 2012

Friday, December 14, 2012

THREE EQUATIONS - THREE UNKOWNS

.....

***************************

In Solving Algebraic Systems we frequently
MULTIPLY an EQUATION by a NUMBER
that will create OPPOSITE COEFFICIENTS.
Be VERY CAREFUL to MULTIPLY
both sides of the EQUATION by the number.
As can be seen in the first example below,
we MULTIPLY equation #1 by (-3)
so that the VARIABLE "P" will cancel.
Notice that we MULTIPLY ALL TERMS BY (-3)
5M(-3) is -15M .... 3N(-3) is -9N ... 1P(-3) is -3P ...and...4(-3) is -1

link for help

___________________________________

___

***********************************************

Tuesday, December 11, 2012

Linear Programming

Linear Programming
*****************************************

*****************************************
#3 is worked out in detail BELOW #3

*****************************************

#3 worked out in DETAIL:

********************************************************

****************************************

Friday, December 7, 2012

Graphing Systems of Inequalities

When graphing Systems of Inequalities you are
finding the REGION of the xy-plane that
contains ALL the ORDERED PAIRS that
make BOTH INEQUALITIES TRUE.

***************************************************

**********************Below is EXAMPLE #1******************

***********************Below is EXAMPLE #2******************

************************Below is EXAMPLE #3*******************

************************Below is EXAMPLE #4********************

*************************Below is EXAMPLE #5***************************

*****************************Below is EXAMPLE #6********************

*************************Below is EXAMPLE #7***************************

****************************Below is EXAMPLE #8*********************

************************Below is EXAMPLE #9************************

*********************************Below is EXAMPLE #10*********************

***************************Below is EXAMPLE #11*********************

***************************************************

Tuesday, November 27, 2012

How to solve Systems of Linear Equations

When solving Simultaneous Equations,
there are THREE (3) OPTIONS:
-----------------------------------------
1) the expected answer is AN ORDERED PAIR.
2) Sometimes the two equations form
the SAME LINE. (An infinite number of solutions)
3) Or the two lines are PARALLEL.
- when parallel there is NO SOLUTION.
-----------------------------------------
1) When finding the answer to ONE OF THE VARIABLES,
you will see something like x = a NUMBER.
Remember that that NUMBER COULD BE ZERO.
so when you get x = 0 that just means that
the FIRST MEMBER of the ORDERED PAIR is ZERO.
-----------------------------------------
2) Option #2 will show up when you see a
result that looks like:
5 = 5 or -34 = -34 or 0 = 0
(Note: the 0=0 result can be confused with
option #1 when x=0 appears)
so 0 = 0 means the SAME LINE.
-----------------------------------------
3) Option #3 produces equations like:
5 = 8 or 0 = 15
These obviously impossible equations
show that the LINES ARE PARALLEL.
-----------------------------------------

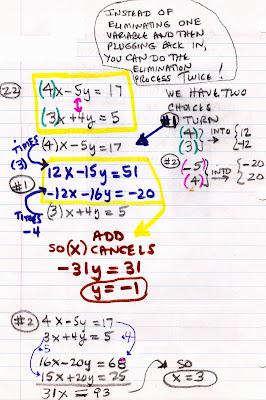
HERE IS THE PROBLEM WE DID TOGETHER

**********************************************************

-----------------------

----------------------------
Example #1 is above
Example #2 is below
-----------------------------

***********************************************
COPY THE TWO PROBLEM
EXAMPLES ABOVE FOR
YOUR QUIZ ON MONDAY.
Put them into your notebook!
*************************************************

.
********************************************